I’ve sung most of my life, yet only recently have I started to ask the question “How do unaccompanied vocalists stay in tune?” The process sounds simple enough at first: vocalists stay in tune because they have good relative or absolute pitch memory — they remember what the 5th of the scale sounds like, and can sing it whenever they like. This explanation works at some level, but a few nagging questions remain.

1. The piano uses Equal Tempered tuning. Natural horns (horns that have no valves) use Just Intonation. What tuning system do unaccompanied voices use?
2. To tune a given chord, which vocalist(s) move, and why?
3. Why do some compositions seem to always go flat or (stranger) sharp, no matter how correctly-tuned each chord and transition is?

To be able to talk about these questions, we need some background information.

The Physical Basis For Western Tuning

Much of the music of the western hemisphere is based on harmonic consonance and dissonance: that two tones sounded together will seem relatively static (consonant) or dynamic (dissonant). This consonance or dissonance is based on the ratio of the frequency (vibrations per second) of one tone to the other.

The ancient Greeks came up with a musical system of tones based on simple ratios. For example, two tones making an Octave have the ratio of 2 to 1, and two tones making a Fifth have the ratio of 3 to 2. The modern western system of tuning has its roots in this ancient Greek tuning system.

In the late 1800’s the physicist Helmholz showed that any periodic sound (like that produced by a musical instrument) can be described as a series of Harmonics (or “Overtones”). The first Harmonic is at the lowest, or Fundamental frequency of the sound; the second harmonic is at 2 times the fundamental’s frequency; the third harmonic is at 3 times the fundamental frequency; and so on.

Helmholz provided a physical explanation for the tuning system of the ancient Greeks. The figure below shows the relationship of the harmonics of a note to the notes of the scale. It also shows several intervals and their relationship to the harmonic series.

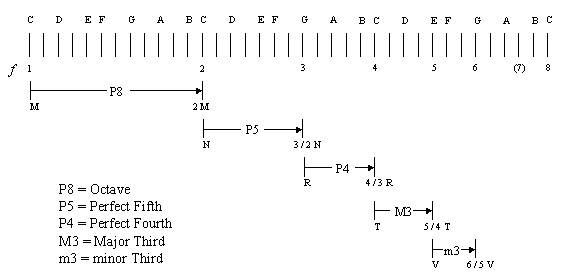
The Octave is the most consonant interval (outside the Unison). It has a ratio of 2 to 1. The Perfect Fifth is next, with a ratio of 3 to 2. Next comes the Perfect Fourth, at the ratio of 4 to 3. Next comes the Major Third, at the ratio of 5 to 4. Finally, the Minor Third has a ratio of 6 to 5.

Tuning Using Beat Frequencies

It turns out that when you hear two tones that are slightly out of tune with each other, you hear them Beat against each other (kind of a “wah wah” effect). There are a number of theories about how the human brain perceives these beats, but the effect is that you actually hear beats at the difference of the frequencies of the two tones. For example, a tone of 440 Hz (cycles per second) sounded at the same time as a tone of 444 Hz produces 4 beats per second – a clearly noticeable difference.

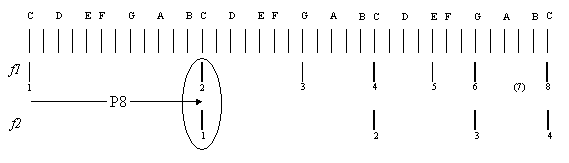
Going back to the harmonic series, we can now show how a pair of vocalists can make very precise adjustments to the pitches they sing. When the pair sings an octave, the second harmonic of one singer is at the same frequency as the fundamental of the other singer. If the two singers are even slightly out of tune, they will hear beats.

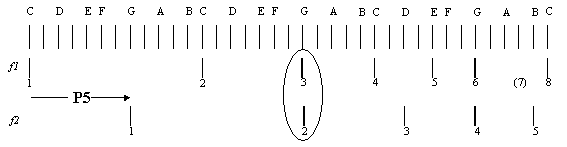
Similarly, when two singers sing an interval of a fifth, the 3rd harmonic of the lower note aligns with the 2nd harmonic of the higher note.

In the interval of the fourth, the 4th harmonic of the lower note aligns with the 3rd harmonic of the higher note.

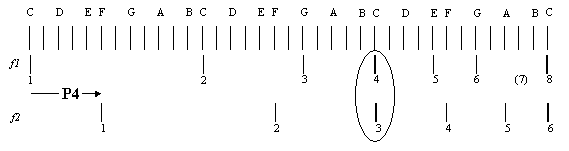
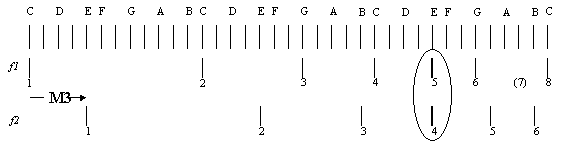
In a major third, the 5th harmonic of the lower note aligns with the 4th harmonic of the higher note.

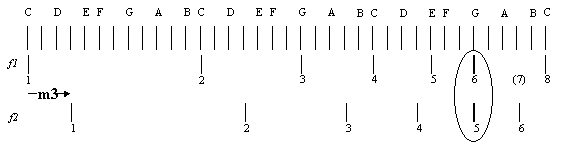
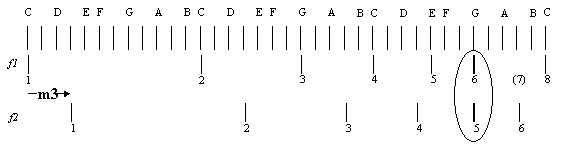
Finally, in a minor third, the 6th harmonic of the lower note aligns with the 5th harmonic of the higher note. The 6th harmonic is clearly audible, it being only two octaves and a fifth above the lower note being sung.

The Problem: Western Tuning Isn’t Perfect

Conventional music of the western hemisphere is based on the 8-tone scale (do re mi fa so la ti do). The trouble is, it’s impossible to construct an 8-tone scale in which all the intervals shown in the figures above are perfectly in tune. The ancient Greek philosopher Pythagorus produced an 8-tone scale based on perfectly-tuned fifths (Pythagorean tuning, named after its inventor), but the thirds are way out of tune. Various musicians over the centuries have wrestled with this problem and proposed tunings that sound better or worse, but a solution remains elusive, if not theoretically impossible.

The modern piano is tuned to a system called “Equal Temperament” or “Equal-tempered tuning”. The big advantage of equal-tempered tuning is that you don’t have to retune your piano to play in a different key: the intervals sound equally out of tune in any key. To put a more positive spin on it, the music sounds equally pleasant in every key.

In Equal Tempered tuning, the Octave is divided into 12 equal semitones. Mathematically, this means that each semitone’s frequency is the 12th root of 2 (or about 1.059463094…) higher than the semitone before it. Each semitone is further divided into 100 equal parts, called “Cents”, allowing tuners to talk about very precise differences in pitch.

Tone	Ratio to C
C	1.0
C# Db	1.05946
D	1.12246
D# Eb	1.18920
E	1.25992
F	1.33484
F# Gb	1.41421
G	1.49831
G# Ab	1.58740
A	1.68179
A# Bb	1.78180
B	1.88775
C	2.0

You can see how far out of tune equal temperament is by comparing it to the pure tunings described above. The octaves, fifths, and fourths are in pretty good shape, but the major thirds and minor thirds are barely tolerable.

Interval	Pure Ratio	Equal Tempered Ratio	Difference (in cents)
Octave	2	2	0
P5	1.5	1.49831	-2
P4	1.33333	1.33484	+2
M3	1.25	1.25992	+14
m3	1.2	1.18920	-16

The Vocalists’ Solution: Change Tuning During the Performance

As I said above, there is no 8-tone scale that will produce pure intervals. Yet an unaccompanied choir does sing pure intervals. The only resolution I can see to this dilemma is that either the choir sings some chords that are noticeably out of tune (unlikely) or they must change tuning during the performance of the piece.

Fortunately, vocalists don’t have to change their tuning much or very often. Although there is no solution for an 8-tone scale, there are a number of “nearly-correct” solutions: scales in which only one interval is badly out of tune.

One such scale is Just Intonation (or “Ptolemaic Temperament“).

Tone	Ratio to C
C	1
D	9/8
E	5/4
F	4/3
G	3/2
A	5/3
B	15/8
C	2

Because the intervals of the Just-tempered scale are not equal, checking the tuning of that scale requires a little more effort than in the equal-temperament case. Here, we need to look at the tuning of each of the chords based on a step of the scale. (I don’t list the vii chord because it’s a very dissonant chord even in the best of cases)

Triad	Notes	M3 ratio	m3 ratio	M3 error	m3 error
I	C E G	1.25	1.2	0	0
IV	F A C	1.25	1.2	0	0
V	G B D	1.25	1.2	0	0
Triad	Notes	m3 ratio	M3 ratio	m3 error	M3 error
ii	D F A	1.18518	1.25	-21	0
iii	E G B	1.2	1.25	0	0
vi	A C E	1.2	1.25	0	0

As you can see, the only bad chord is the ii chord. Traditionally, such a badly out of tune chord is called a “wolf”, because it “howls”. This chord can be repaired by re-tuning the D of the scale from a ratio of 9 / 8 to one of 10 / 9. Of course this change will throw off a lot of the other chords, so the D must be tuned to a ratio of 10 / 9 for the ii chord and 9 / 8 for all other chords.

Alternatively, instead of changing the D of the ii chord, we could change the F and A of that chord.

The Vocalists’ Dilemma: Which Notes to Change During the Performance

Now we’ve come to the interesting question: If unaccompanied vocalists sing perfectly-tuned intervals, and if the ii chord of the scale must take on two different tunings, which of the two choices above (change the D vs. change the F and A) does a choir take during the performance?

Since changing the tuning of a sustained note is very noticeable (and difficult to do precisely), I suspect that vocalists tend to follow just one rule: If two chords in a progression share a tone, keep the tuning of that shared tone constant — that is, tune the other notes in the second chord to the tone that is in common with the first.

Now all I have to do is examine some traditional vocal music and see what happens to the tuning during the piece if I follow the rule above.

Wish me luck!

For Further Reading

• Terry Blackburn‘s music page describes the Pythagorean error (the “comma”), and graphically compares various historical tuning systems.
• The Medieval Music & Arts Foundation has a good description of gothic polyphony.